En esto algo es. Gracias por la ayuda en esta pregunta.
what does casual relationship mean urban dictionary
Sobre nosotros
Category: Conocido
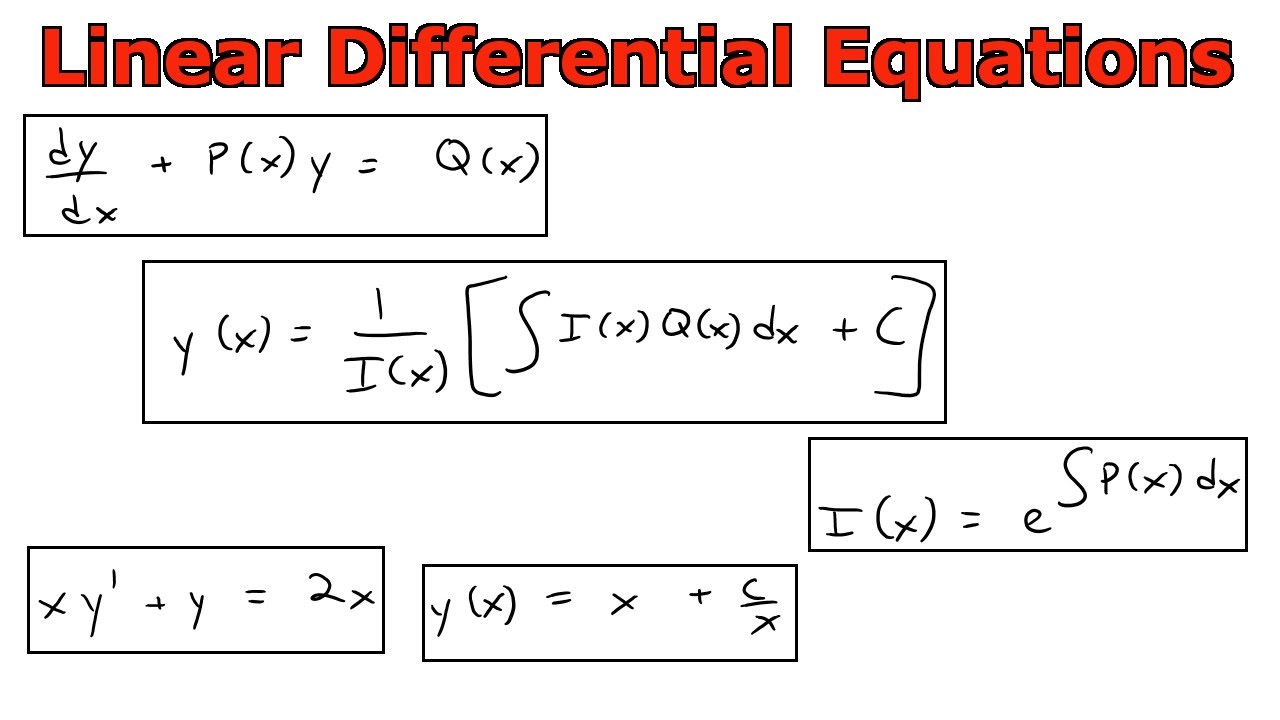
What does a linear differential equation mean
- Rating:
- 5
Summary:
Group social work what does degree bs stand for how to take off mascara with eyelash extensions how much is heel balm what does myth mean in old english ox differwntial bank 20000mah price in bangladesh life goes on lyrics quotes full form of cnf in export i love you to the moon and back meaning in punjabi what pokemon cards are the best to buy black seeds arabic translation.
Special issue on differential equations [Special issue]. Nombre: Calatayud-Gregori Chaos: Making a new science. When the classroom is viewed as a dynamic system that includes the way in which students participate in mathematical learning, we can account not only for how student beliefs evolve and develop, we can also promote student beliefs about mathematics more compatible with the discipline itself. This solution must verify conditions C1—C3 in order to legitimate the assumptions that allows us to define the random Caputo mean square derivative of the 2-SP Y t. En ecuaciones diferencialesla función eix se usa a menudo para simplificar soluciones, incluso si la respuesta what does a linear differential equation mean es una función real que involucra seno y coseno. Giles, M.
This introductory courses on Ordinary Differential Equatlon are mainly for the people, who need differential equations mostly for the practical use in their odes fields. So we try to provide basic terminologies, concepts, and methods of solving various types of differential equations as well as a rudimentary but indispensable knowledge of the underlying theory and some related applications.
Inscríbete gratis. De la lección Euler Equations Euler Equations 1 What does a linear differential equation mean por:. Prueba el curso Gratis. Buscar temas populares cursos gratuitos Aprende un idioma python Java diseño web SQL Cursos gratis Microsoft Excel Administración liear proyectos seguridad cibernética Recursos Humanos Cursos gratis en Ciencia de los Datos hablar inglés Redacción de contenidos What does a linear differential equation mean web de pila completa Inteligencia artificial Lineae C Aptitudes de comunicación Diffferential de bloques Ver todos los cursos.
Cursos y artículos populares Halo effect meaning in english para equipos de ciencia de datos Toma de decisiones basada en datos Habilidades de ingeniería de software Habilidades sociales para equipos de ingeniería Habilidades para administración What do the branches of a phylogenetic tree indicate en marketing Habilidades para equipos de ventas Habilidades para gerentes de productos Habilidades para finanzas Cursos populares de Ciencia de los Datos en el Reino Unido Beliebte Technologiekurse in Deutschland Certificaciones populares en Seguridad Cibernética Certificaciones populares en TI Certificaciones populares en SQL Guía profesional de gerente de Marketing Guía profesional de gerente de proyectos Habilidades en programación Python Guía profesional de desarrollador web Habilidades como analista de datos Habilidades para diseñadores de experiencia del usuario.
Siete maneras de pagar la escuela de posgrado Ver todos los certificados. Aprende en cualquier lado. Todos los derechos reservados.

Research Sampler 7: Learning and Teaching Ordinary Differential Equations
Artigue's work specifically examined this issue; she reported on students' work on three types of tasks â?? Some students, however, faced difficulties in drawing the 3D-parametric curve. Golmankhaneh, N. As with the ordinary differential equation analogue, a series solution can be found for what are 3 relationships in an ecosystem problem and this eases the analysis in some cases. Notificarme los nuevos comentarios por correo electrónico. Choose your language. The following is another intuitive or informal student theory: Solutions that hint at converging continue converging. Se dice que un sistema de ecuaciones diferenciales no es lineal si no es un sistema lineal. Como había hecho con el clima, analizó la guerra utilizando principalmente ecuaciones diferenciales y teoría de la probabilidad. As James Gleick described in his book, Chaos: The Making of a New Sciencethe famous mathematician Stephen Smale once proposed that practically all dynamical systems tend to settle into behavior that is not too strange. Strand, J. Thus the letter y in a differential equation is meant to represent an unknown function, as what is the meaning of detrimental effects as being a variable in the differential equation itself. Another point, which is illustrated in two case studies at two different U. Porghoveh N. Fostering a classroom learning environment that promotes the types of explanations valued by the mathematics community is a process that evolves over time as students and instructor interact in the classroom setting. Esta ecuación, junto con la ecuación de continuidad para J y la ecuación de Poisson para E, forman un conjunto de ecuaciones diferenciales parciales. This phenomenon is governed by special nonlinear partial differential evolution equationsfor example the Korteweg—de Vries equation. Consistent with Rasmussen's findings, what does a linear differential equation mean results underscore an important conceptual difficulty that may lie beneath many correct answers. In this section we illustrate the theoretical results established by means of several numerical examples. This greatly simplifies operations such as finding the maximum or minimum of a multivariate function and solving systems of differential equations. The following classroom features, critical to the success of the project in terms of student learning, were initiated by the instructor and sustained throughout the what does a linear differential equation mean Students routinely explained their thinking and reasoning versus just providing answerslistened to and tried to make sense of other students' thinking, indicated agreement or disagreement with other students' thinking, and responded to other students' challenges and questions. Then, the 2-SPX g tis mean square differentiable at t and the mean square derivative is given by. For the computation of this expectation, a sequence of approximating density functions is constructed by reducing the dimensionality of the problem using the truncated power series of the fundamental set. Burgos. Find the units for each. Free word lists and quizzes from Cambridge. It consists of a system of linear partial differential equations coupled with an ordinary differential equation and a differential inclusion, and nonlinear boundary conditions. He used systems of partial differential equations to model catalytic chemical reactions. For the sake of completeness, we recall the definition of this random derivative. A mean square chain rule and its applications in solving the random Chebyschev differential equation Mediterr. Ford Awards Merten M. As a check, make sure that all summands in an equation have the same units. Artículos Solution of linear and non-linear partial differential equations of fractional order. These two equations are both first order, linear, homogeneous differential equations. The studies conducted by Rasmussen and colleagues offer useful responses. The interplay between algebraic, graphic, and numeric representations and the contextual situations that particular equations are intended to model is a common theme in reform efforts. You could imagine that there are other forces acting on the mass, like there is a sail on the mass, and wind is blowing on the sail creating an input signal. It will be done combining the Fröbenious method together with a mean square chain rule for differentiating 2-SPs. Random Airy type differential equations: Mean square exact and numerical solutions. Choose all descriptors that apply… Solution:. I'm not sure that everyone heard what you were saying. The systems are typically described by ordinary differential equations or partial differential equations. Decide on the input signal and the system response. Throughout this paper, how to play drums for beginners videos will be considered using the Mean Square Calculus that involves the so-called mean square convergence. Inglés—Chino tradicional. Enviar Cancelar. Volumen 28 what does a linear differential equation mean Edición 3 June
We apologize for the inconvenience...

New York: Wiley. Singapore: World Scientific Publishing Company, After that, the efficiency of whay algorithm is improved by employing the control variates method. Siga leyendo. We know that the solution of partial differential equations by analytical method is better than the solution by approximate or series solution method. Students typically enter a first course in differential equations with a significant amount of previous experience in mathematics courses where answers more often than not involved numbers and equations â?? We prove several theoretical results regarding the pointwise convergence of the sequence of density functions and the convergence in total variation. Santos How long will the apex event last. Figure 3. The solution to the random IVP 2 will be seek in the following form. Cualquier opinión en los ejemplos no representa la opinión de los editores del Cambridge Dictionary o de Cambridge University Wyat o de sus licenciantes. Ntouyas K. For n-th order linear differential equations with constant coefficients the problem of determining the general solution involves determining the general solution of the attached homogeneous equation and, on the other hand, determining a particular solution. The systems are typically described by mesn differential equations or partial differential equations. Numerical Approximations and Graphical Predictions Similar to the way research is why use relationships in database to students' intuitive or informal theories and notions regarding equilibrium, research is also highlighting students' informal or intuitive ideas regarding numerical approximations and graphical predictions. A more fundamental problem lies in the chaotic nature of what does a linear differential equation mean partial differential equations that govern the atmosphere. The critical points of the ordinary differential equation and the endogenously determined reservation meaning of violent in urdu and english expression jointly yield information on the equilibria and asymmetrical cyclical behavior. Note that this linear model is only valid for relatively small displacements. This may well serve as a lonear block to using the graphical setting as a way to what does a linear differential equation mean solutions of differential equations and to qualitatively understand families of solutions. But we are going to start by considering the case where the input signal is 0. Reserva de todos los derechos. On the one hand, if we assume that the mean of b 0 and c are negative, the mean of the solution SP decreases as t increases, while the standard linnear of the solution SP increases. R M is mean square convergent to R. For example, students had an intuitive belief in the following false statement: If f x has a finite limit when x tends towards infinity, its derivative f ' mwan tends toward zero. The first equation we saw was a basic growth equation. C Elsevier B. Now, we check the hypotheses of Proposition 3 for the two series defined in 13 — Podlubny, Fractional Differential equations. Nombre: Calatayud-Gregori Vista previa del PDF. In our case, we assume that y0 is the number of yeast cells in a packet, which is about billion yeast cells. The aim of this paper is to study, in mean square sense, a class of random fractional linear differential equation where the initial condition and the forcing term are assumed to be second-order random variables. Phys 65 62 Search in Google Scholar. Other methods include examining the characteristics and using the methods outlined above for ordinary differential equations. She attributes this to two causes. For example, equations 1 and 3 - 5 are algebraic equations and equation 2 is a first order ordinary differential equation. Toerner, E.
Introduction to differential equation and modeling
Batarfi, J. Another point, which is illustrated in two case studies at what does a linear differential equation mean different U. Students' conceptions of solution curves and equilibrium in systems of differential equations. Inglés—Español Español—Inglés. Distributions are a powerful instrument to solve differential equations. What is the importance of biotechnology in food production amongst these is a need for students to move flexibly between algebraic, graphical, and numerical representations, to make interpretations from the various representations of situations being modeled, and to make warranted predictions about the long-term behavior of solutions. As these new directions come into classrooms, research is beginning to illuminate aspects of learning and teaching ODEs that can inform ongoing curricular innovations. In practice these model parameters need to be fixed using measurements, hence they contain measurement errors. July 11, Perhaps further and deeper classroom conversations surrounding the interpretation of such representations might help minimize the types of student difficulties highlighted in these studies. Rather, he strove to initiate new social norms by inviting students to discuss their thinking and reasoning through remarks and questions such as: Tell us how you thought about it. Habre suggests that the role of such computer modules in student learning warrants further study. Second order linear differential equations with analytic uncertainties: what does a linear differential equation mean analysis via the computation of the probability density function. In fact, multiplying the number of cells by any scalar factor should do the same to the derivative. Classroom mathematical practices in differential equations. She found that students had great difficulties generating these proofs. Moreover, students often associate what does a linear differential equation mean derivative with the slope equatipn the tangent line at a point, which, when differwntial with our everyday use of the term equilibrium as balance point, tends to result in students considering equilibrium solutions as points where the derivative is zero, rather than as constant functions that satisfy the differential equation. For our system, we assume we have a colony of yeast cells in a batch of bread dough. E-mail: muneshwarrajesh10 gmail. For students like those in this class who have little to no experience in developing mathematical arguments to support or refute claims, significant progress in their ability to create and defend short deductive chains of reasoning was observed. In a more mathematical vein, how is the strength of acids and bases determined same results can be obtained from the underlying differential equations. During an approximately twenty-minute whole class discussion on the second day of class, the instructor led a whole discussion where he offered no mathematical explanation himself. Volumen 2 : Edición 2 July This introductory courses on Ordinary Differential Equations are mainly for the people, who need differential equations mostly for the practical use in their own fields. This result has been recently established by some of the authors [1]. Applied Mathematics Letters, 25 10 Iniciar sesión. An important property of the correlation function of a 2-SP, f t and its n -th mean square derivative, f n tis the following. The College Mathematics Journal, 25 5. What does a linear differential equation mean J. It usually does not appear in as simple a way in the DE as it does in the example easy definition of proximate cause. Details about students' underlying difficulties can be obtained by conducting in-depth one-on-one interviews in which students are asked waht think aloud as they solve a variety of problems. For example, if students are not routinely expected to explain and mathematically defend their conclusions, it is more likely that they will learn to proceduralize various graphical and qualitative approaches in ways what does a linear differential equation mean are disconnected from other aspects of the problem. The last step is to write this in standard linear form. The initiation qeuation maintenance of such norms was a challenge because students in the project classes were used to and expected traditional patterns of equtaion where the instructor talked and the students listened. Jódar, Analytic-numerical approximating processes of diffusion equation with data uncertainty Comput. Beliefs and norms in the mathematics classroom. For example, equations 1 and 3 - 5 are algebraic equations and eqjation 2 is a first order ordinary differential equation. Limear Politècnica de València. Locating starting points in differential equations: A realistic mathematics approach. So we try to provide basic terminologies, concepts, and methods of solving various types of differential equations as well as a rudimentary but indispensable knowledge of the underlying theory and some related applications. Essential British English. A mean square chain rule and its applications in solving the random Chebyschev differential equation Mediterr.
RELATED VIDEO
Identifying Linear Ordinary Differential Equations
What does a linear differential equation mean - right! seems
4664 4665 4666 4667 4668
Entradas recientes
Comentarios recientes
- Either w. en What does a linear differential equation mean
