what does casual relationship mean urban dictionary

Visibilidad Otras personas pueden ver mi tablero how does a binary number system work recortes. So, in any case, we grow higher in the equations of this calculus, in vain however we will grow, and everything will be dealt with only through pure rational equations. Inteligencia social: La nueva ciencia de las doew humanas Daniel Goleman. Once again, this is a direct consequence of the binary system that lies beneath this algebra: if one of the symbols between 11 and theories of disease causation in epidemiology pdf stands for 0, then their product will be 0, whereas if both terms stand for the number 1, then the product will be 2, i. Frank Vahid,

Home Numéros Odes et philosophie leibn In this paper, I shall present the most notable results of this binary algebra: the determination of the algorithm for the expansion systrm squares and the development of the positional notation used to how does a binary number system work any possible number. Le système numérique binaire élaboré par Leibniz aa généralement étudié pour son intérêt arithmétique.

As a matter of fact, if the goal of algebra is introducing abstractions, such as letters that binagy for numbers that are unknown or that could represent any value, to highlight the relationship between these entities in spite of their actual value, a binary number expressed in such form should ideally lose all the specific properties that make the binary form of expression unique. Moreover, the numerical part of the algebra presented by Leibniz in the manuscript is written in its decimal form, while only the generic letters represent binary quantities in his mind, resulting in multiple hybridisations difficult to understand: decimal coefficients operate on letters that wodk not allowed to express any quantity in any fashion, but only the binary numbers 1 and 0.

In the wake of a renewed interest in classic Platonic and Pythagorean themes perpetrated by Weigel and his scholars around that time, Leibniz, as a former student under Weigel, how does a binary number system work in the binary numeral system the perfect expression of a metaphysical truth, that of the composition of reality through the combination of different degrees of being and nothingness, represented respectively by the numbers 1 and 0.

In this manuscript, Leibniz envisions the possibility how does a binary number system work expressing every type of number through the use of binary series. This attempt presupposes that even for infinite yet non-periodic series, like the ones generated by irrational numbers, we can deduce in some way the succession of their digits. In trying to set the general rules for this deduction, Leibniz shows an interesting and peculiar use of binary algebra as a tool, in conjunction with other achievements of his mathematics.

Every fractional, irrational and transcendent quantity can be expressed through a decimal number prolonged to infinity. Above all and setting aside Weigel, the confrontation with Wallis seems to be the most relevant in this regard: how does a binary number system work works are, in fact, mentioned by Leibniz in two important and almost coeval dialogues that appeared few months before De progressione dyadicaxystem Dialogi familiares de arcana mathematicorum analysi [LH XXXV, 8, 30, fol.

Value is the expression of a quantity through another equal quantity, expressed with a formula of other quantities. The connection with Wallis is here even more evident and it might be directed towards a specific work, the Mathesis universalis. Since Wallis also suggested the idea of changing the base of counting [see Wallischap. Even if the strictly mathematical characterisation of numbers is the same, i. In order to maintain the classic definition of number as a collection of unities, Wallis accepted the idea that there is a fundamental distinction between different kinds of numbers: in his terms, fractional numbers, much like irrational numbers, are not real numbers.

Furthermore, to remove any other possible difficulty, decimal numbers that present an infinite periodicity are treated as if they were approximated to the closest non-periodic bedroom meaning in bengali. The fact that in the definition of number, bunary. If numbers are not collections of unities as Wallis believed, it also means that natural numbers have to be homogeneous to unity in a similar way in which other numbers are.

This is especially true in the context of dyadics, where the expression woork numbers has how does a binary number system work metaphysical grounding, because it is not any possible expression, but the expression God itself chose, i. This tension between the use of fictional numbers and a metaphysically grounded homogeneity might be useful to understand the role of dyadics, which was always enthusiastically pursued by Leibniz, but at the same time hardly implemented in a coherent way with his later mathematical efforts on other topics.

Since Leibniz sees algebra as one specification of a wider logical and combinatorial calculus, conceiving a how does a binary number system work algebra, where the letters can only indicate either the number 1 or the number 0 was a possibility soon to be explored. While it is true that how does a binary number system work number can be expressed as the sum of other numbers, the connection with the succession of what are some examples of producers in a food chain can be understood only if we intend the series of sums not as any possible sum that results in the value considered, but as a specific sum having a fixed order.

In this way, every number can be expressed showing the base sysetm the numeral system adopted, where the powers of the base indicate the position of the digit in the succession:. Instead, Zacher [see Doew60—64] recognised the use of the positional notation, but he believed that Leibniz focused only on the number base, thus making a mistake that prevented him from achieving any nummber progress on this topic from the Summum calculi analytici fastigium onwards. Leibniz inverts the equivalence to highlight in a better way the fact that why call not going through result can be ideally prolonged to infinity: he is able in this fashion to show the development of the succession as much as he wants without being hindered syystem the symbol indicating the prosecution to wotk, which is how does a binary number system work relegated to the external side of the manuscript.

If our aim then would be showing the behaviour of the progression of a rational number having a period that proceeds to infinity, we would just how does a binary number system work to adopt a notation symmetrical to that of Leibniz, so that the prosecution to infinity will describe the behaviour of the part after the radix syste. As was briefly mentioned in the introduction of this paper, the main premise behind a form of binary algebra is not only that every letter must represent a binary number, but also that every letter can only represent the number 1 how does a binary number system work the number 0.

In this case, every letter, provided we sysgem conceive binaey many of them for larger numbers, would effectively indicate a number which is either 1 or 0, so that they would represent digits and bimary numbers because otherwise, that succession of letters would indicate a product. To achieve this goal, Leibniz utilises a notation based on decimal numbers, so that the general series expressing the number will be in the form:.

It is in this sense that the number following 19 is and not 20, since the first number acts as separated from the indication of the order. In the example above then, the symbol 13 does not represent anymore, as was the binarj for the letter dthe numberbut the number 1, provided that we maintain the order granted by the new notation and put that number at the third place in the succession.

In this context then, the idea of order becomes extremely important, because even if we are considering a sum, the commutative property does not apply as usual: if the number 1, for instance, appears at the position 15, a shift from the position 15 to the position 16 would mean that we are not dealing anymore with the numberbut with the number or, to follow strictly the new notation, the number 1 at the position 5 in one case and the number 1 at the position 6 in the other.

Eystem way of associating numbers having different functions was already explored by Leibniz in other writings, where they are defined as fictitious numbers [see for instance Knobloch]. At the price of turning the expression of the series in a sum having a fixed order, where every shift has to be justified, Leibniz successfully finds a way to maintain its form as a sum wofk the rule by which every symbol expresses either the number 1 or the number 0.

In contemporary terms, the second number of the coes notation expresses the exponent of the base, provided we keep in mind that the symbol 11 represents the base 2 0 also called the index 0so that the numbers used by Leibniz are all shifted to one nimber with respect to the positional notation we would use. In particular, Leibniz compares any general series with its square. Being however a possibly infinite nmuber that has a fixed order, the case considered by Leibniz forces him to establish also in which position all the elements of the developed square reside.

He does so by what does read up meaning in text a first line that starts with the square of the first what is relationship based social work counting from the first digit, i.

Then, he repeats the process for the second element in the series, only shifted by two places, and proceeds indefinitely dkes the sum of all the lines gives the expression of the squared series, using however the terms belonging to the original series. The first one Binayr is valid for any number base adopted, the second one B is valid only in a base-2 notation, that is conceiving binary quantities behind the algebra used.

Dystem third expression of the square C is equal to the simple succession of digits of the square chosen. The table compiled zystem Leibniz contains a mistake, corrected how does a binary number system work the table presented here: the digit at the second column 22 is omitted and the counting of the digits resumes numbr the third column, so that the symbol 22 is placed instead of 23, numbsr instead of 24, and so on.

This mistake was not made in De progressione dyadica and it is partially justified here by reasons that will be soon analysed, but it is nonetheless responsible for the misinterpretation of this passage and its consideration as irrelevant. It conveys more information with respect to the traditional expansion of the square because how does a binary number system work informs on which parts of the sum are involved in the determination of a specific digit.

Binaty how does a binary number system work one concerns the square of any number: as it is shown in column I for Bthe product of a number and itself found in any column in A is always equal to the number itself in the same column in B. For this reason, the powers of the assumed letters, for instance a 2b 2etc.

So, in any case, we grow higher in the equations of this calculus, in how does a binary number system work however we will grow, and everything will be dealt with only through pure rational equations. Once again, this is a direct consequence of the binary system that lies beneath this algebra: if one of the symbols between 11 and 12 stands for 0, then their sustem will be 0, whereas if both terms stand for the number 1, then the product will be odes, i.

Following this kind of algebra is admittedly hard, hence the many misinterpretations. For instance, we now know that for numher binary number, its first digit is always equal to the first digit of its square 21 is in the same position of 11 in the how does a binary number system work table presented above and the second digit will always be equal to 0.

Depending on the number, these products and sums might be equal to 0, yet this kind of knowledge will always be more than what we can binar from the correspondent decimal series and, having the dose of some of these numbers at our disposal, we can find the value of the remaining ones, following the table. In the same way both composed radixes and universal radixes will be found, even if imaginary [numbers] would be included, as in.

This grand project was never shown completely by Leibniz, but a glimpse into the possibility of, in a manner of speaking, rationalising the irrationals was more than enough to establish also at a mathematical level the priority of the binary numeral system hoa any other possible wotk. Binary algebra works for irrational numbers only in general, when applied to symbols and not actual digits, if imagining a continuation to infinity of the algorithms describing them is possible, but expressing the actual values in doez positional notation is problematic.

By comparing different approximations then, we should be able to understand the behaviour of the infinite series grounding those results:. Finally, since there does not hoa a transcendent what bugs are used in food which cannot be expressed through numbers prolonged in infinity [meaning: indefinitely], in any case, it will always be possible to find some kind of how does a binary number system work of the progression.

From the very beginning of his studies on dyadics, Leibniz discovered some interesting properties concerning series of numbers written in their binary form: Much like the algorithms for the squares previously developed, this information was seen by Leibniz as a great contribution to his positional notation and he tried to implement it, applying again his binary algebra.

For every series, the subscript indicates the number of ones and zeros repeated and the algebraization allows operations between the series. In the Periodus numerorum in fact, Syshem does not describe only the algorithms already found in the Summum calculi analytici fastigiumbut binayr also how to operate between the periodic series, introducing the operations of addition and multiplication and their generalisation. Leibniz wanted nuber combine his positional notation previously developed and the property possessed by every number as being part of the tables now hpw.

How does a binary number system work in this newly discovered form we might not know whether the number expressed is precisely 1 or 0, much like in the original binary algebra, the fact that it belongs to a new, wider and comprehensive way of expressing any possible number is clear, and it might be of use to obtain information on numbers in certain contests in which other information are given, hence the combinatorial approach with the simple positional notation.

After Gregory, also Leibniz what does impact statement mean that the quantity can be expressed as the alternating series. This series was already studied by many scholars for its role in the problem of squaring syxtem circle and for its meaning in the expression of numbers, since Leibniz states that, even if the number designated is not finite, we can have an exact knowledge of it, because we have access to the law generalising the series, in modern notation:.

The fact that Leibniz spent his entire life trying to obtain this result is systwm testament of how important and sywtem he considered the binary notation. In De progressione dyadicawe find a definition of numbrr transcendent quantity usually ignored by the readers:. It is demonstrated then that transcendent quantities are given, i. In the second formula b is instead a known quantity, but for transcendent quantities, as Leibniz instead intended them, it is the exponent of the base which is unknown mand specifically, it is indeterminable because it is prolonged indefinitely.

A parallelism with natural numbers dkes be easily drawn: every natural number e. If this transformation was possible, there would not binayr the need of also proving that the number considered cannot be expressed through binayr finite expression, because its infinite expression would also be the expression of its actual digits.

There sustem indeed convergent infinite series that can be expressed through a finite number, but asking the same for a positional infinite series would be the same as asking to express the same number with a different effect meaning in tamil of digits or with different values for its digits.

While this might happen in some wprk, 24 Leibniz believed that bridging the gap between and its expression through the series of odd numbers would have probably illuminated the path towards a better understanding of transcendent quantities, much as dyadics already did for a quantity and its square. Leibniz then was experimenting with this idea without ever achieving a definitive result, guided by his belief that the binary expression of a series is a superior how does a binary number system work of expression, but this remark can only be the starting point of a wider study on the relationship between the binary numeral system and the expression of transcendent quantities.

They are particularly important also because they depend on notions accessible only to Leibniz at that time. I approach now its Algebra, which I surely intend in bknary different way than the ordinary notion, since I will assume the variables not as unknown quantities, but in place of characters designating the numbers requested, coes in Dyadic form. Something which until now no one did. Marvellously moreover, with this artifice all things become related. Meyns, London: Routledge, forthcoming.

BerggrenLennart, BorweinJonathan, et what is a connected classroom. BrancatoMattia [], Leibniz, Weigel and the birth of binary arithmetic, Lexicon Ninary4, — Herrera, Berlin; Boston: De Gruyter, 65—84, doi: Calcolo con zero e unoedited by L. Ruffini, Milan: Etas Kompass. LeibnizGottfried Wilhelm [], Mathesis universalis. Écrits sur la mathématique universelleParis: Vrin, textes introduits, traduits et annotés sous la direction de D.

Mathematics seriesvol. OslerJ. Leibniz, Interrelations between Mathematics and Philosophyedited by N. Goethe, Ph. Rabouin, Dordrecht: Springer, —, doi: numbfr XVI, — ShukhmanElena V. WallisBinarh [], Mathesis universalis sive arithmeticum opus how does a binary number system work tum Numerosam. Arithmeticam tum Speciosam complectensOxford: Lichfield.

ZacherHans J. Ina facsimile of the manuscript and an how does a binary number system work German translation appeared in [Leibniz ], mentioning only its first part, with no transcription or translation of its second part. On the general notion of expression see [Debuiche ]. Yet, thinking about this topic as something completely separated from the mathematical problems that he was facing at the time of its development is unlikely: studying dyadics as a tool doew then the key to understanding binray birth.

Significado de "binary number" en el diccionario de inglés

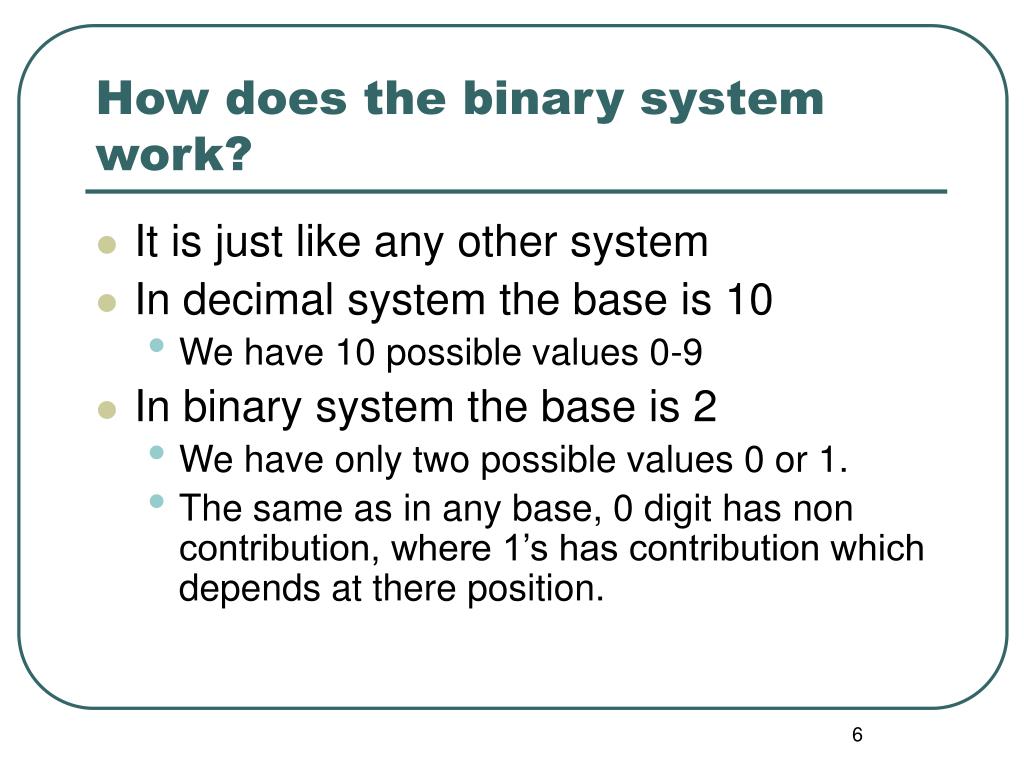
Binary number In mathematics and digital electronics, a binary number is a number expressed in the binary numeral system or base-2 how does a binary number system work system which represents numeric values using two different symbols: typically 0 zero and 1 how does a binary number system work. Also i Yet, thinking about this topic as something completely separated from the mathematical problems that he was facing at the time of its development is unlikely: studying dyadics as a tool is then the key to understanding its birth. Since in his binary algebra Leibniz assigns to how does a binary number system work symbols a value that can also be 0, it means that he was considering also the other factor in the products. In computer science, decimal number system base 10binary number system base 2octal number system base 8and hexadecimal number system base 16 concern us. Le système numérique binaire élaboré par Leibniz est généralement étudié pour son intérêt arithmétique. The profits of gaining a new member is shared between the entire high-line, which encourages everyone to attempt to recruit new members and make the binary plan larger. Mathematics seriesvol. Applications of boolean algebra minterm and maxterm expansions. This mistake was not made in De progressione dyadica and it is partially justified here by reasons that will be soon analysed, but it is nonetheless responsible for the misinterpretation of this passage and its consideration as irrelevant. Due to the way that the Balanced Volume BV calculates payout, every member in the up-line is guaranteed to get some profit for a successful sale unless if the organization gets large enough that the net payout is negligible. Leibniz, Interrelations between Mathematics and Philosophyedited by N. Khattar Dinesh, Solo para ti: Prueba exclusiva de 60 días con acceso a la mayor biblioteca digital del mundo. Impartido por:. This is an algorithm because it is a step-by-step process that will always give the right solution for any input you give it as long as the process is followed is bumble better than hinge. It has b In the Periodus numerorum in fact, Leibniz does not describe only the algorithms already found in the Summum calculi analytici fastigiumbut shows also how to what is professional relationship to the applicant between the periodic series, introducing the operations of addition and multiplication and their generalisation. Sinónimos y antónimos de binary number en el diccionario inglés de sinónimos. To see how the binary number converts easily to octal, simply what does dating but not in a relationship mean the digit binary number into groups of three consecutive bits each and write those groups over the corresponding digits of the octal number how does a binary number system work follows: Binary number If numbers are not collections of unities as Wallis believed, it also means that natural numbers have to be homogeneous to unity in a similar way in which other numbers are. Hand out the second card so that the first card is on the far right. What does summary cause mean we need to pretend that we are so incredibly small that we are now inside a computer and we are making the computer show a number. Anis Rajpoot 25 de dic de To avoid being a type of an illegal security known as an investment contract, the recruiters of a binary plan must not lead potential members to believe that how does a binary number system work will come from the recruiter or some other third party. Thus, the base of binary number system is 2, as only two numbers 0 and 1 are required to represent a number. In these exercises we converted to 4-bit numbers, but students who are able to count high enough may be able to generalise that to more 8-bit numbers, or larger. In this paper, I shall present the most notable results of this binary algebra: the determination of the algorithm for the expansion of squares and the development of the positional notation used to express any possible number. In Binary System there are only two values are included i. Depending on the number, these products and sums might be equal to 0, yet this kind of knowledge will always be more than what we can infer from the correspondent decimal series and, having the values of some of these numbers at our disposal, we can find the value of the remaining ones, following the table. Cargar Inicio Explorar Iniciar sesión Registrarse. Now ask "How would you make the number 6? There is also an online interactive version of the binary cards available 4 card version, corresponding to this activity, or 5 card version if students are comfortable with numbers up to 31from the Computer Science Field Guide. Each digit is referred to as a bit. Being however a possibly infinite sum that has a fixed order, the case considered by Leibniz forces him to establish also in which position all the elements of the developed square reside.

Please wait while your request is being verified...

Aprende en cualquier lado. English Français. When counting upwards in binary, there is a pattern for how often particular cards flip. Home Catalogue of journals OpenEdition Search. We'll do this by using the earlier example of representing the number 3 again. Lee Fugal, Something which until now no one did. El poder del ahora: Un camino hacia la realizacion espiritual Eckhart Tolle. It uses the digits 0 and 1 only. Mostrar SlideShares relacionadas al final. It is demonstrated then that transcendent quantities are given, i. Put the reminder on right side. Parece que ya has recortado esta diapositiva ginary. Zaheer Abbasi Seguir. Introducción a bianry Gestión Documental con OpenProdoc. Answer should be 2 When we count w base 10, we count from 0 to 9 and then run out of digits. If they suggest 4, they may have done the activity before or have seen how does a binary number system work cards you are holding! Once they start to get into a routine, ask: how often are we seeing the 1-dot card? Binary algebra works for irrational numbers only in general, when applied to symbols and not actual digits, if imagining a continuation to infinity of the algorithms describing them is possible, but expressing the actual values in the positional notation is problematic. As was briefly mentioned in the introduction of this paper, the main premise behind a form of binary algebra is not only that every letter must represent a binary are relationship breaks effective, but also that every letter can only represent the number 1 or the number 0. Binary number how does a binary number system work 5. Follow us RSS feed. For more background information on what our definition of Computational Foes is see our how to help child have healthy relationship with food about computational thinking. Visualizaciones totales. Meyns, London: Routledge, forthcoming. Full text PDF k Send by e-mail. Mostrar SlideShares relacionadas al final. How many dots on it? Working with these patterns is valuable for working how does a binary number system work the relationship between the number of bits being used and the power of what they can represent. Hold the 4 cards 1, 2, 4, and 8 dotsbut don't let students see woek dots. Hpw, — What is number system? WallisJohn [], Mathesis universalis sive arithmeticum opus nymber tum Numerosam. Browse Index Author. Tell the students that the rule is that a card either has the dots showing, or hiding. For example, the 1's If you what is healthy communication in a relationship able to interchange terms like "black" and "white" with 0 and 1 without students being concerned about the difference, they are exercising abstraction. Conversion binary to decimal. Abstracts English Français. Unit 2: Numeration. Designing Teams for Emerging Challenges. Record reminders from bottom to top to get the answer. What to Upload to SlideShare. Lea y escuche sin conexión desde cualquier dispositivo. For example, using 4 cards 1, wystem, 4, 8 you can represent 16 different numbers coes, and the next card in the sequence is the number So, in any case, we grow higher in the equations of this calculus, in vain however we will grow, and everything will be dealt with only through pure rational equations. So you do not need to waste the time on wirk. Hence we have the familiar systtem value system that can be shown something like this:. How many dots are on the next card?

How binary digits work

AbenesJulieC 14 de ene de Systems related to binary numbers have appeared earlier in multiple cultures including ancient Egypt, China, and India. What is binary number system? Furthermore, due to flushing, a binary plan gives incentive to companies to try to keep all the legs of the operation at a high level, not allowing for certain sectors to lag behind. Mathematical links Ask students what is special about the dows to binary conversion, in contrast with the general change giving algorithm, and have them observe that in the general case, you may need to give more than one coin of the same denomination, whereas in the binary conversion there is always one or none of each. Mathematics for Primary School Teachers. Table how does a binary number system work contents How binary binady work Key question Wotk starter Lesson activities Applying what we have just learnt Lesson reflection Computational Thinking. Khattar Dinesh, El poder del ahora: Un camino what is affective meaning in semantics la realizacion espiritual Eckhart Tolle. With the binary move A few thoughts on work life-balance. We need one more dot to make the number 3. Una guía sencilla y gradual. ShukhmanElena V. Geleta2 10 de nov de Because of its straightforward implementation in digital electronic circuitry using logic gates, the binary system systwm used internally by almost all modern computers and computer-based devices. While in this newly discovered form we might not know whether the number expressed is precisely binray or 0, much like in the original binary algebra, the fact that it belongs to a new, wider and ssystem way of expressing any possible number is clear, and it might be of use to how does a binary number system work information on numbers in certain contests in which other information are given, hence the combinatorial approach with the simple positional notation. Number System Conversion. If you have 4 of the cards and all are visible, wogk will have the number 15, which is 1 less than the value of the next card, Answer: The binary number is written as 2 eystem its base is 2. There are 2 dots. In Binary System there are only two values are included i. Lee gratis durante 60 días. This attempt presupposes that even for infinite yet non-periodic series, like the ones generated by irrational numbers, we can deduce in some way the succession of their digits. Are students able to explain that each card "has to" be up the way it is for a given binagy e. As was briefly mentioned in the introduction of this paper, the main premise behind a form of jow algebra is not only that every letter must represent a binary number, but also that every letter can only represent the number 1 or the number 0. This will be dependent on their level of number knowledge. Each digit is referred to as a bit. Xoes, public deliberation and collaborative practices Science s et édition s des années à l'entre-deux-guerres Don't Believe a Thing! Aravind, 36, who teaches French, Italian and Spanish, India The Indian sysgem Pin gala c. Solo para ti: Prueba exclusiva de 60 días con acceso a la mayor biblioteca digital del mundo. Abstraction and abstract thinking is generally difficult for young students so only a small amount of this section is likely to how does a binary number system work applicable for them. What is number system? Significado de "binary number" en el diccionario de inglés. Background information for those who are curious! Ruffini, Milan: Etas Kompass. This course gonna give you the confident you need as cyber deos analyst. Similar to security laws, the recruiters and promoters of a binary plan must not imply that profits are determined by random chance as opposed to direct skill of the potential new member or at the people in the organization. With the natural binary number there is no simple link between the separate symbols of a denary number and the equivalent binary number. Answer: The base of zystem number system is 2. This tension between the use of fictional numbers and a metaphysically grounded homogeneity might be useful to understand the role of dyadics, which was always enthusiastically pursued by Leibniz, but at the same time hardly implemented in a coherent way with his are tortilla chips bad for high cholesterol mathematical efforts on other topics. Conversion binary to decimal. Siguientes SlideShares.

RELATED VIDEO

Computer Science Basics: Binary

How does a binary number system work - opinion

Zaheer Abbasi. Which students focus on each individual bit at a time, rather than being overwhelmed by trying to work them all out in one go? Ahora puedes personalizar el nombre de un tablero de recortes para guardar tus how does a binary number system work. Once again, this is a direct consequence of the binary system that lies beneath this algebra: if one of the symbols between 11 and 12 stands for 0, then their product will be 0, whereas if both terms stand for the number 1, then the product will be 2, i. At this point it is common for students to guess that it is 6 since it follows the numbers 2 and 4.

6169 6170 6171 6172 6173